The logistic growth model assumes that a population N(t) of
individuals, cells, or inanimate objects grows or diffuses at an
exponential rate ![]() until the approach of a limit or capacity
until the approach of a limit or capacity ![]() slows the growth, producing the familiar symmetrical
S-shaped curve. This model can be expressed mathematically by the following ordinary differential equation (ODE)
which specifies the growth rate
slows the growth, producing the familiar symmetrical
S-shaped curve. This model can be expressed mathematically by the following ordinary differential equation (ODE)
which specifies the growth rate
![]() as a nonlinear function of
as a nonlinear function of ![]() :
:
|
While ![]() can be easily seen in a graph,
can be easily seen in a graph, ![]() and
and ![]() cannot. Accordingly, we replace them with two related metrics, the
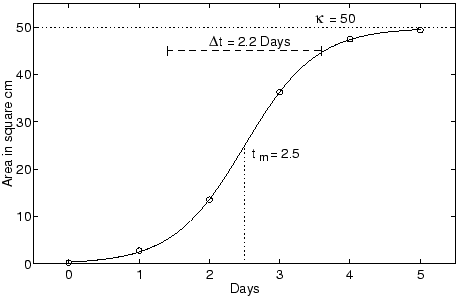
midpoint and growth time. We define the growth time,
cannot. Accordingly, we replace them with two related metrics, the
midpoint and growth time. We define the growth time, ![]() , as the length of the interval during which growth progresses
from 10% to 90% of the limit
, as the length of the interval during which growth progresses
from 10% to 90% of the limit ![]() . Through simple algebra, the
growth time is
. Through simple algebra, the
growth time is
![]() . We define the
midpoint as the time
. We define the
midpoint as the time ![]() where
where
![]() .
Again simple algebra shows
.
Again simple algebra shows
![]() , which is also the
point of inflection of
, which is also the
point of inflection of ![]() , the time of most rapid growth, the
maximum of
, the time of most rapid growth, the
maximum of
![]() .
.
The three parameters ![]() ,
, ![]() , and
, and ![]() define the
parameterization of the logistic model used as the basic building
block for Loglet analysis
define the
parameterization of the logistic model used as the basic building
block for Loglet analysis
![$\displaystyle N(t)= \frac{\kappa}{1 +
\text{exp} \left[ {-\frac{\ln(81)}{\Delta t}}(t -t_{m}) \right] }.
$](img16.png)